Copyright © Karl Dahlke, 2023
At an amusement park, a fun house typically contains curved mirrors that distort your image in unusual ways. As a thought experiment, assume every wall in a fun house is a mirror. Walls could be straight or curved, but every wall is a mirror. Everywhere you look you see yourself, or perhaps hundreds of images of yourself if mirrors stand directly across from each other.
Shine a flashlight directly into a wall, and the light beam bounces straight back to you. If you point your flashlight at an angle, the beam of light bounces all around the house faster than you can imagine. In fact it probably makes its way into every nook and cranny.
Assume the fun house is closed to the outside world, so that light never enters or leaves the house. Yet inside, none of the rooms are closed off. In other words, every room has an open door leading to another room. The interior forms a connected set. There is one light in the house. When it is turned on, does light spread throughout the entire house, or is there a small room somewhere that remains dark?
If all the walls consist of straight lines, we're pretty sure the entire house is lit. However, if some of the walls are curved, e.g. circular arcs, then some of the rooms might remain dark. How is thiis possible?
Here is a simple solution, though it is not very practical. Place the light at the center of your picture and draw a circle of radius 1 around it. Then draw another circle of radius 2 concentric with the first circle. In other words, the light is at the center of both circles. Beams of light leave the center, bounce straight back from the inner circle, and return to the center. The light shines on itself. The outer room, the annulus between the two circles, remains dark, but that's because it is closed off. Cut a small door in the inner circle. Light now streams out through this door, bounces off the outer circle, and returns to the center. As long as you're not standing near the doorway, the outer room remains dark.
This works in theory, but not in practice. If a person or object is placed in the inner circle, some of the light bounces off that object and leaves the inner room at a different angle. This light does not bounce straight back into the inner room. Instead it bounces away and spreads throughout the annulus. The slightest speck of dust or imperfection in the mirror spreads light throughout the house. There is a better design that works in the real world. It could be turned into a functioning science exhibit. People can stand in one room, well lit, and then step into another room in complete darkness, even though the walls are all mirrors.
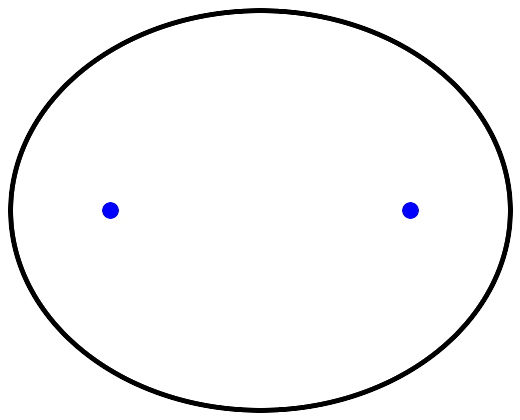
First, some background about light, mirrors and curved surfaces. Imagine a circle with a dot at the center. As mentioned above, a light ray that leaves the dot, in any direction, bounces off the mirror and returns to the dot. Now stretch the circle, left to right, so that it becomes an ellipse. At the same time, separate the dot into two dots, pulling them apart as you stretch the ellipse. Each dot is a focus of the ellipse, and the two dots together are called foci, the plural of focus.

Assume the ellipse is a mirror, just as the circle was a mirror. A light ray that leaves either focus bounces off the mirror and goes to the other focus. That's why it is called a focus, the focus of the light. Place a light at one focus, and all its energy is sent towards the other focus. The behavior of this mirror, and other curved mirrors, was determined by Isaac Newton, as he constructed the first reflecting telescope.
Spin the ellipse around its major (longest) axis to get a three dimensional ellipsoid. It looks a bit like an American football with rounded ends. Again, all the light from one focus bounces over to the other. Imagine such an ellipsoid a billion miles across and three billion miles long. Put the sun at one focus and yourself at the other. All the energy of the sun, from every square meter of its surface, bounces off the mirror and onto you. As the old Cheetos commercial use to say, you would be “quick fried to a crackly crunch.”
Here is a more pleasant experiment. Stand at one focus, while your girlfriend stands at the other. The ellipse could be the size of a room, or a baseball stadium, or the solar system. You will see your girlfriend everywhere you look. If you and she are standing back to back, then you can see the back of her head reflected in the curved mirror in front of you. Turn to the left, and you will see her right ear coming into view, and then her profile, as though you were walking around her. At 180 degrees about you can see a giant image of her face in the far mirror, perhaps interrupted by the back of her head, which you see directly. Keep turning to see the other side of her face, her left ear, and then the back of her head once again. At every angle it's her, nothing but her, and at every angle she sees you, and nothing but you. You also hear each other, speaking in a whisper, even if you are hundreds of meters apart. Sound can bounce off of walls just like light.
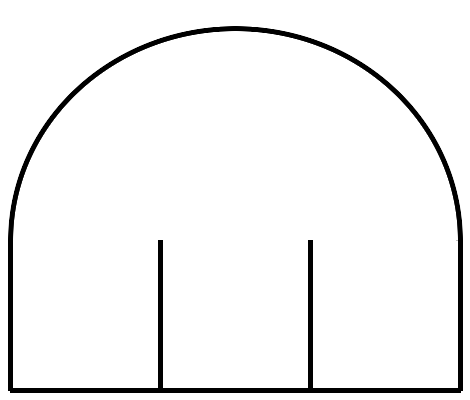
Now we're ready to build my fun house. Near the bottom of your paper, draw a horizontal line 6 inches long. Then draw four vertical line segments 2 inches high, standing up from your horizontal base at 2 inch intervals. You have built three rooms, each room 2 inches square.
Now draw the top half of an ellipse, joining the two outer walls. This closes off the house. Let the two foci of the ellipse be the top end points of the two inner walls. Call these points a and b. If a beam of light leaves point a, it is leaving one focus of the ellipse. It bounces off the curve of the ellipse and returns to b. Similarly, any light leaving b bounces off the curved mirror and returns to a.
Suppose a light ray leaves the middle room at a point p, somewhere between a and b, traveling in any direction. Let this light ray strike the elliptical mirror at a point c. In other words, light travels along the segment pc. Draw the lines ac and bc, light bouncing from one focus to the other. pc lies inside ∠acb. Remember that the angle of incidence equals the angle of reflection. pc strikes the mirror at an angle that is truer, i.e. closer to the perpendicular, than ac, thus the reflected ray is truer then cb. The reflected ray lies inside ∠acb, and returns to a point q somewhere between a and b. Put this all together and any light leaving the middle room returns to the middle room. If the light is on in the middle room, the left and right rooms remain dark. This holds true even if people and furniture are in the middle room, and scatter the light in different directions.
If this were an actual exhibit in a science museum, I think visitors would be amazed. Stand in the middle room and you can see everything, the furniture, your friends, your own reflection if you look directly into one of the walls. Walk around to one of the outer rooms, and as soon as you step inside it is pitch dark. You blink your eyes, straining for any light at all. I suppose some will leak in, but it's pretty dog gone dark. You walk back around to the middle room and all is bright again.
Alternatively, place the light in the left room, and it will bounce over to the right room and back again, leaving the middle room in darkness. For a great effect, place a red light in the middle room and a green light in the left room. Visitors will see either a green world or a red world, depending on which room they are standing in. Their eyes don't have to adjust to light and dark, eliminating more than a few liability concerns. They can tell, by the change in color, and by the images in the mirrors, that the rooms are optically separated, though the mechanism of separation is not obvious.
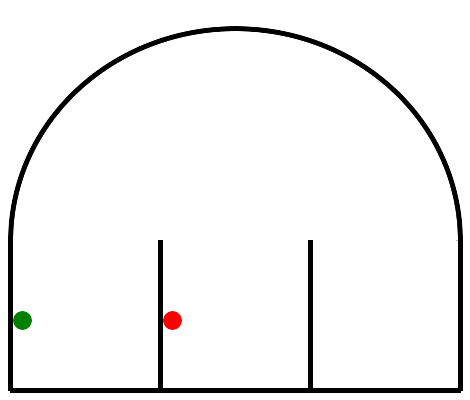
If a person steps into the elliptical hallway, he scatters light in all directions, and all three rooms are lit. The hallway must be free of people and objects, and the mirrors must be near perfect.
This design is my own idea; please contact me if you want to use it for a science exhibit, or any other purpose. Here is some math on Conic sections and mirrors.